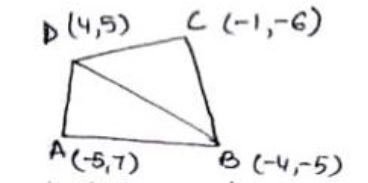
If A(-5, 7), B(-4, -5), C(-1, -6) and D(4, 5) are the vertices of a quadrilateral
Coordinate Geometry (10)If A(-5, 7), B(-4, -5), C(-1, -6) and D(4, 5) are the vertices of a quadrilateral, find the area of the quadrilateral ABCD.
Answer
Area of quad ABCD = Ar ΔABD + Ar ΔBCD
Area of ΔABD = $\frac{1}{2}$ |(-5)(-5 - 5) + (-4)(5 - 7) + (4)(7 +5)|
= 53 sq units
Area of ΔBCD = $\frac{1}{2}$ | (-4)(-6-5)+ (-1)(5 +5)+4(-5+6)|
= 19 sq units
Hence, area of quad. ABCD = 53 + 19 = 72 sq units
Exam Year:
2018
Related Questions
- Write the coordinates of a point P on x-axis which is equidistant
- If the distances of P(x, y) from A(5, 1) and B(-1, 5) are equal
- If A(-2, 1), B(a, 0), C(4, b) and D(1, 2) are the vertices of a parallelogram ABCD
- Use of mobile screen for long hours makes your eye sight weak and give you headaches
- Find the ratio in which P(4, m) divides the line segment joining the points A(2, 3)
- In what ratio does the point (24/11, y) divide the line segment joining the points P(2, - 2) and Q(3, 7)