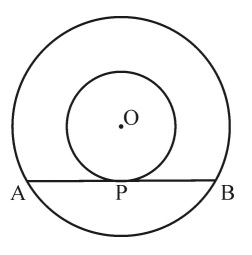
Two concentric circles with centre O are of radii 3 cm and 5 cm
Circles (10)Two concentric circles with centre O are of radii 3 cm and 5 cm. Find the length of chord AB of the larger circle which touches the smaller circle at P.
Answer
Join OA and OP
OP ⊥ AB (radius ⊥ tangent at the point of contact)
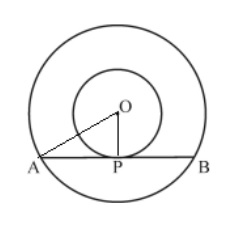
OP is the radius of smaller circle and AB is tangent at P.
AB is chord of larger circle and OP ⊥ AB
∴ AP = PB (⊥ from centre bisects the chord)
In right Δ AOP, AP2 = OA2 – OP2
= 52 - 32 = 16
AP = 4 cm = PB
AB = 8 cm
- Exam Year: 2023
Related Questions
- How many tangents can be drawn to a circle from a point on it
- The length of the tangent from an external point A to a circle
- The length of the arc of a circle of radius 14 cm which subtends an angle of
- Prove that the tangents drawn at the end points of a chord of a circle make equal angles with the chord
- In the given figure, XY and X'Y'are two parallel tangents to a circle with centre O
- In the adjoining figure, PT is a tangent at T to the circle with centre O. If $ \angle TPO = 30^o $, find the value of x